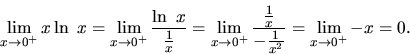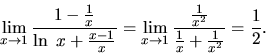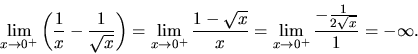Nech
-
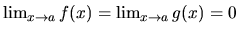 ,
,
- v istom okolí čísla
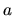 majú obidve funkcie
majú obidve funkcie 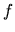 a
a 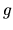 deriváciu,
deriváciu,
- existuje
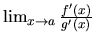 .
.
Potom existuje aj
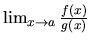 a platí
a platí
Užitočnosť tohoto pravidla vynikne ešte viac, ak si uvedomíme, že
limity "typov
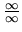 ,
, 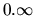 ,
,
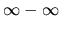 ,
,
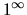 ,
, 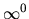 ,
, 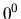 " môžeme pomocou úprav previesť na
limitu "typu
" môžeme pomocou úprav previesť na
limitu "typu 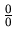 ".
".
Príklad 27.
Pomocou L'Hospitalovho pravidla vypočítame niekoľko
limít. Pri počítaní každej limity samostatne overte predpoklady
použitia pravidla.
Riešenie:
Typ 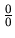 :
:
Typ
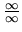 :
:
Typ 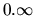 :
:
Niekedy používame L'Hospitalovo pravidlo viackrát za sebou.
Typ
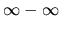 :
:
Typ 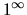 : (použijeme rovnosť
: (použijeme rovnosť
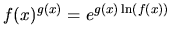 )
)
Pozor na nesprávne použitie L'Hospitalovho pravidla:
Nájsť chybu v tomto postupe a opraviť ju necháme na čitateľa.
![]() :
: