Asymptotou grafu funkcie je priamka, ktorá sa grafu funkcie "dotýka
v nekonečne". Rozoznávame dva druhy asymptôt grafu funkcie.
Priamka 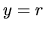 je asymptotou grafu funkcie
je asymptotou grafu funkcie 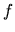 bez smernice,
ak funkcia
bez smernice,
ak funkcia 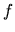 má v bode
má v bode 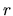 (aspoň jednostrannú) nevlastnú
limitu.
(aspoň jednostrannú) nevlastnú
limitu.
Priamka  je asymptotou grafu funkcie
je asymptotou grafu funkcie 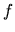 so smernicou,
ak
so smernicou,
ak
![$\lim_{x \rightarrow \infty} [f(x) - (px+q)] = 0$](img2502.gif) alebo
alebo
![$\lim_{x \rightarrow -\infty} [f(x) - (px+q)] = 0$](img2503.gif) .
.
Pre praktické zisťovanie asymptôt grafu funkcie platia pravidlá:
- Priamka
 je asymptotou grafu funkcie
je asymptotou grafu funkcie 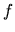 bez smernice,
ak aspoň jedna z limít
bez smernice,
ak aspoň jedna z limít
 alebo
alebo
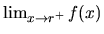 je rovná buď
je rovná buď 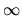 alebo
alebo
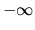 .
.
- Priamka
 je asymptotou grafu funkcie
je asymptotou grafu funkcie 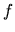 so smernicou, ak
so smernicou, ak
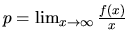 a
a
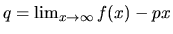 .
.
Príklad 30.
Nájdime asymptoty grafov funkcií
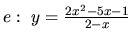 ,
,
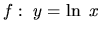 ,
,
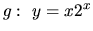 ,
,
 ,
,
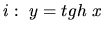 ,
,
Riešenie:
Funkcia  nie je definovaná v jedinom reálnom čísle
nie je definovaná v jedinom reálnom čísle 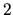 , preto
jediná možná asymptota bez smernice je priamka
, preto
jediná možná asymptota bez smernice je priamka 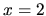 .
Počítajme
.
Počítajme
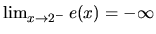 , preto spomínaná
priamka je asymptotou grafu
, preto spomínaná
priamka je asymptotou grafu  . Ďalej
. Ďalej
(prečo?) a
Priamka 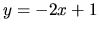 je asymptotou grafu
je asymptotou grafu  .
Tá istá priamka je aj asymptotou grafu
.
Tá istá priamka je aj asymptotou grafu  v
v 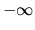 (overte!).
Poznamenajme, že asymptoty racionálnych funkcií môžeme počítať aj
jednoduchšie prepísaním funkcie pomocou delenia mnohočlenov:
(overte!).
Poznamenajme, že asymptoty racionálnych funkcií môžeme počítať aj
jednoduchšie prepísaním funkcie pomocou delenia mnohočlenov:
Z tohoto zápisu je vidieť, že priamka 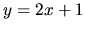 je jedinou asymptotou
grafu
je jedinou asymptotou
grafu  .
.
Funkcia 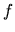 je spojitá a
je spojitá a
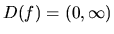 . Naviac
. Naviac
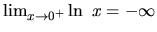 , preto priamka
, preto priamka 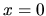 je
asymptotou grafu
je
asymptotou grafu 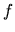 . Ďalej
. Ďalej
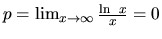 avšak
avšak
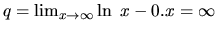 . Preto
funkcia
. Preto
funkcia 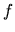 nemá asymptotu so smernicou.
nemá asymptotu so smernicou.
Funkcia 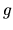 je spojitá v každom reálnom čísle, preto nemá
asymptoty bez smernice. Ďalej
je spojitá v každom reálnom čísle, preto nemá
asymptoty bez smernice. Ďalej
nie je vlastná a preto 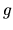 nemá asymptotu v
nemá asymptotu v 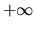 .
Na druhej strane
.
Na druhej strane
a
(prečo?). Priamka 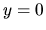 je asymptotou grafu funkcie
je asymptotou grafu funkcie 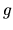 .
.
Funkcia 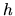 nie je definovaná v číslach
nie je definovaná v číslach
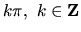 a vo
všetkých okrem
a vo
všetkých okrem  má nevlastné jednostranné limity (overte!).
V čísle
má nevlastné jednostranné limity (overte!).
V čísle  je
je
Preto funkcia 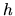 má nekonečne veľa asymptôt bez smernice určených
rovnicami
má nekonečne veľa asymptôt bez smernice určených
rovnicami
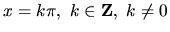 .
Funkcia
.
Funkcia 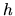 osciluje pre
osciluje pre
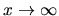 aj pre
aj pre
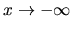 , preto nemá asymptoty so smernicou.
, preto nemá asymptoty so smernicou.
Funkcia 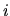 je spojitá vo všetkých reálnych číslach, preto nemá
asymptoty bez smernice. Ďalej
je spojitá vo všetkých reálnych číslach, preto nemá
asymptoty bez smernice. Ďalej
pretože 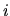 je ohraničená funkcia a
je ohraničená funkcia a
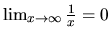 .
Potom
.
Potom
Priamka 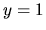 je asymptotou grafu funkcie
je asymptotou grafu funkcie 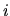 . Pretože funkcia
. Pretože funkcia 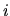 je nepárna, je asymptotou aj priamka
je nepárna, je asymptotou aj priamka  (odôvodnite!).
(odôvodnite!).
Príklad 31.
Ukážeme, že, ak má párna funkcia asymptotu  ,
tak má aj asymptotu
,
tak má aj asymptotu 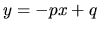 .
.
Riešenie: Pretože graf párnej funkcie je symetrický podľa osi 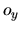 ,
z predpokladu asymptoty
,
z predpokladu asymptoty  vyplýva aj existencia asymptoty
súmerne združenej s ňou podľa osi
vyplýva aj existencia asymptoty
súmerne združenej s ňou podľa osi 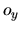 . Touto je priamka
. Touto je priamka 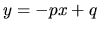 (overte!).
(overte!).
![]() ,
,
![]() ,
,
![]() ,
,
![]() ,
,
![]() ,
,
![]() nie je definovaná v jedinom reálnom čísle
nie je definovaná v jedinom reálnom čísle ![]() , preto
jediná možná asymptota bez smernice je priamka
, preto
jediná možná asymptota bez smernice je priamka ![]() .
Počítajme
.
Počítajme
![]() , preto spomínaná
priamka je asymptotou grafu
, preto spomínaná
priamka je asymptotou grafu ![]() . Ďalej
. Ďalej
![]() ,
tak má aj asymptotu
,
tak má aj asymptotu ![]() .
.
![]() ,
z predpokladu asymptoty
,
z predpokladu asymptoty ![]() vyplýva aj existencia asymptoty
súmerne združenej s ňou podľa osi
vyplýva aj existencia asymptoty
súmerne združenej s ňou podľa osi ![]() . Touto je priamka
. Touto je priamka ![]() (overte!).
(overte!).
![]()